American Scientist

ONCE UPON A PRIME: The Wondrous Connections Between Mathematics and Literature. Sarah Hart. 304 pp. Flatiron Books, 2023. $29.99.

The Commonwealth of Mathematics and the Republic of Letters share a long, unguarded border. Conventional wisdom says there’s no need to fortify the boundary line, because so few citizens of either realm have any inclination to cross it. It’s not that math types and literary types are enemies; perhaps they are merely indifferent to each other’s ideas.

In Once Upon A Prime, Sarah Hart reveals that there is more cross-border traffic than most of us realize. Various kinds of mathematical contraband have been smuggled into the works of poets and novelists, and at least a few mathematicians have dabbled in works of imaginative literature. Hart wants to encourage such cultural commerce, and perhaps even establish more formal diplomatic relations.

Once Upon A Prime offers a three-part catalog of literary works that borrow from the world of mathematics in one way or another. In Part I, mathematical ideas supply patterns and structures that give shape to poetry or to works of prose fiction. An example is the Japanese art of haiku, poems with 17 syllables arranged in three lines of five, seven, and five. Hart points out that 3, 5, 7, and 17 are all prime numbers, divisible only by 1 and themselves. The Japanese tanka form has five lines with syllable counts of five, seven, five, seven, and seven; the total is 31, which is another prime. This prevalence of primes is intriguing, although Hart offers only tentative speculations about its effect on the aesthetics of the poems.

The rhyme schemes of poems also have a mathematical structure. A rhyme scheme can be represented by a sequence such as ABAB or ABBA, where matching letters indicate lines with the same end rhyme. A natural question for those with a mathematical bent is how many rhyme schemes are possible for verses with a given number of lines. The answer is a sequence of numbers beginning 1, 2, 5, 15, 52, 203. . . . That is, a one-line poem has just one possible rhyme scheme, A. With two lines the alternatives are AA and AB. Three-line verses have these five possible schemes: AAA, AAB, ABA, ABB, ABC. The elements of the sequence grow rapidly for longer verses; with 10 lines, there are 115,975 possibilities.

Hart explains that the numbers counting rhyme schemes are known as Bell numbers, after the mathematician Eric Temple Bell. To my disappointment, however, she does not explain in any detail why this particular sequence is associated with the rhyme schemes, or how to calculate the Bell numbers.

The celebration of mathematically inspired literary structure reaches its zany zenith in the works of Oulipo, a group formed in 1960 by the French writers Raymond Queneau and François Le Lionnais. “Oulipo” is an abbreviation of Ouvroir de littérature potentielle, usually translated as Workshop of Potential Literature. A characteristic work from the group is Queneau’s One Hundred Thousand Billion Poems, published as a book of 10 pages. Each page is slit horizontally to form 14 strips, and each strip bears a single line of text. Selecting one of the 10 strips in each of the 14 positions generates the promised 10¹⁴ poems. They are all sonnets conforming to the same rhyme scheme. American mathematics writer Martin Gardner claimed they all make sense, although he can’t possibly have read them all.

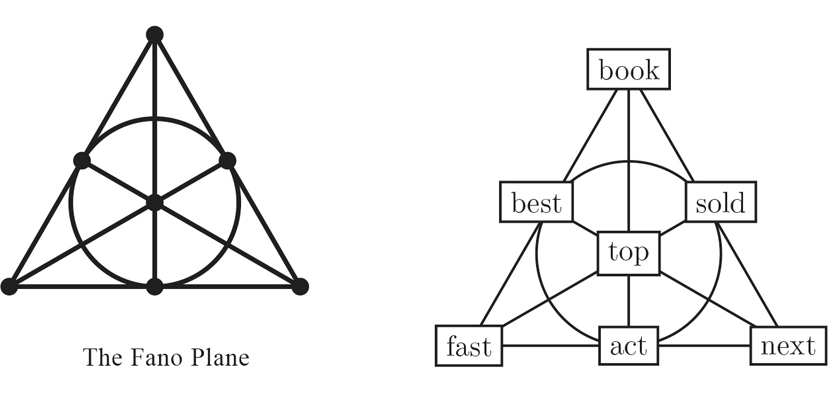
Hart herself bravely enters the potential-literature game with a device she calls Fano fiction. The Fano plane, named for the Italian mathematician Gino Fano, is a geometric construction with seven points and seven lines, arranged as shown above. Each point lies on three lines, and each line touches three points. (One of the lines is drawn as a circle; no arrangement of the points allows all seven lines to be drawn as straight segments.) By associating a word with each point, Hart generates a story made up of seven three-word sentences, beginning “Book top act! Best book fast!” The story consists of 21 words, but explaining what they mean would take at least four times as many.

Part II of Once Upon A Prime explores mathematical ideas that enter literary works as metaphors or other figures of speech. Hart describes her experience of reading Herman Melville’s Moby-Dick for the first time:

As I read on, I kept encountering mathematical allusions, so many in fact that it became clear to me that Melville obviously relished mathematical ideas—they were bound to escape from his mind onto the page, and when he reached for a metaphor, more often than not something mathematical would present itself.

The premier example is Melville’s description of iron cauldrons called try-pots aboard the whaling ship Pequod. These large cooking vessels, where whale blubber was rendered into oil, have a cross section that the narrator, Ishmael, identifies as an inverted cycloid. The cycloid is the curve traced by a point on the perimeter of a disk as the disk rolls on a plane. Ishmael, having climbed into one of the pots to scrub and polish its interior, muses on the geometry of the surface: “It was in the left hand try-pot of the Pequod, with the soapstone diligently circling around me, that I was first indirectly struck by the remarkable fact, that in geometry all bodies gliding along the cycloid, my soapstone for example, will descend from any point in precisely the same time.”

The cycloid curve was a hot topic among mathematicians in the 17th century, when Christiaan Huygens proved the “remarkable fact” noted by Ishmael: The inverted curve is a tautochrone (a term that might be translated “same time”). A body sliding along the curve without friction will reach the bottom in the same amount of time, no matter where along the curve it is released. It’s surprising that Ishmael, a sailor with little formal education, would be acquainted with such a geometric oddity, but then Ishmael is quite the know-it-all, discoursing on everything from ancient history to zoology.

A more pointed question is how Melville came to know of the cycloid and the tautochrone. Hart has an answer to propose: He learned it from Joseph Henry, one of the preeminent American scientists of the 19th century. Henry’s first teaching appointment was at the Albany Academy, a school for boys where young Melville was a star pupil. This aspect of Melville’s biography was brought to light in a 2016 doctoral thesis by Meredith Farmer, who argues that Melville’s interest in the sciences was not merely ignored, but had been actively suppressed by some biographers.

Part III discusses works in which mathematics (and in some cases, mathematicians) become central, thematic elements of the story. For example, fractals and chaos theory, both trendy mathematical topics in the late 20th century, were important plot devices in Michael Crichton’s novel Jurassic Park. Cryptography was a theme in works by Edgar Allan Poe, Jules Verne, and O. Henry. We visit Professor James Moriarty, the archvillain of the Sherlock Holmes stories, whose professorship is in mathematics. Hart points out that Holmes himself, with his skills in logical deduction, might have been given mathematical credentials, but Arthur Conan Doyle thought the public would have trouble warming up to such a figure. Better an opium addict than a mathematician.

The longest section of Part III delves into Edwin A. Abbott’s 1884 book Flatland: A Romance of Many Dimensions. This is a work with a highly mathematical premise: Various creatures inhabiting a two-dimensional universe try to imagine life in three dimensions, thereby challenging us denizens of 3D to conceive of a fourth dimension. There’s no disputing that mathematical ideas take center stage in this tale. Indeed, it’s really more of a math book than a novel, and library catalogs treat it as such, putting Flatland on the mathematics shelves, not with the fiction.

For me, the most engaging section of Part III deals with Hart’s attempt to resolve a seeming paradox in a Jorge Luis Borges short story, “The Library of Babel.” The library holds a single copy of every possible book of a certain length; each volume consists of 410 pages, holding 1,312,000 characters drawn from an alphabet of 25 letters and punctuation marks. Thus, the library’s total inventory is 25^1,312,000 books, which is an immense number, but still finite. The paradox is that the library itself is said to go on forever, with every shelf filled. You can walk left or right in any corridor and never come to the end, and likewise, you can go up or down stairways from floor to floor without reaching the top or the bottom. How can we reconcile this boundless space with a finite collection of volumes? Hart proposes that we map the corridors and stairways onto the surface of a torus, or doughnut. That way, you can wander forever without coming to the edge of the world.

By education and profession, Hart is a member of the mathematical community, rather than the literary one. She is a professor of mathematics at Birkbeck College in London and is also the current Gresham Professor of Geometry, a post that calls for delivering a series of lectures for the general public. She is a talented and enthusiastic expositor, who clearly takes great pleasure in turning people on to the charms of mathematics. It’s a little perplexing, then, that in this book she sometimes shies away from fully explaining the mathematical ideas she discusses.

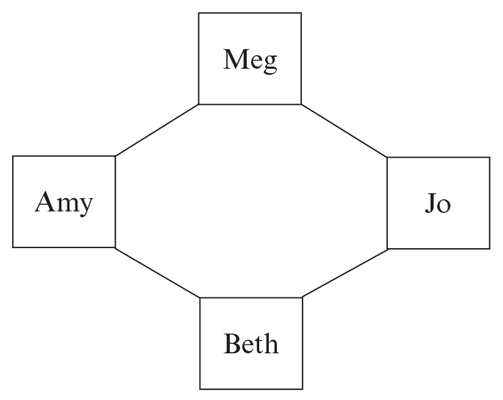
As mentioned, the Bell numbers and rhyme schemes receive only cursory treatment. Another example comes up in connection with a structure called an interval graph. Hart introduces the idea with a story about the four sisters of Little Women visiting their Aunt March. The interval graph records these visits by drawing a line between two sisters if their visits overlapped. Reports from Meg, Jo, Beth, and Amy lead to the interval graph shown above. Hart then writes:

This graph has a cycle Meg—Jo—Beth—Amy. But there is a theorem in graph theory that says that every interval graph is ‘chordal.’ What this means is that somewhere in every cycle there has to be a chord—an intermediate line joining two of its points. If a graph doesn’t have that property, then it can’t be a true interval graph. Here, it means there must be a line either joining Meg to Beth, or one joining Amy to Jo.

I was fascinated by this observation, but also frustrated that Hart gave no hint of why the theorem is true. Perhaps she chose not to spell out the details for fear of scaring away math-shy readers. Hart writes, “That feeling we get when we read a great novel or a perfect sonnet— that here is a beautiful thing, with all the component parts fitting together perfectly in a harmonious whole—is the same feeling a mathematician experiences when reading a beautiful proof.” Agreed! And the reader should not be denied a taste of the delights to be found in reading proofs and other kinds of mathematical literature.