The Soap Film: An Analogue Computer
By Cyril Isenberg
Soap films provide a simple method of obtaining analogue solutions to some mathematical problems
Soap films provide a simple method of obtaining analogue solutions to some mathematical problems

DOI: 10.1511/2012.96.243

Editors’ Note: This Classic article was first published in the September–October 1976 issue, and has been reprinted as part of American Scientist’s centennial-year celebrations. The author based his article on a popular lecture-demonstration given during his visit to American universities. Dr. Isenberg, who remains at the University of Kent since the writing of this article, still gives his soap-film demonstrations about 50 times a year, to audiences that range from young people to members of learned societies. In 2008, Queen Elizabeth II made him a member of the Most Excellent Order of the British Empire for his contributions to physics. Reprints of letters in response to the original printing of this article are available at the end of this article. Other articles on soap films and mathematics have appeared in American Scientist in May–June 1986, September–October 1996 and March–April 2000. The latter is available online: "Foams and Honeycombs."
The advent of digital computers in the 1950s and their rapid growth in the 1960s and 1970s has resulted in the neglect of analogue computers and analogue methods. Most analogue computers are designed to solve specific problems and are usually only capable of two-figure accuracy. Digital computers do not have these limitations, but analogue computers usually have one advantage: they provide a speedy visual solution to a problem. One of the simplest and most impressive analogue methods is based on a physical property of soap films, and in this article I will show how these methods can be used to solve mathematical minimization problems.
A well-known principle in thermodynamics states that, at constant temperature, the free energy of a system will be minimized when it is in thermodynamic equilibrium. The system may consist of a fluid, a solid, a gas, or combinations of different substances in these three phases. In a system consisting of a soap film, this free energy, F, is proportional to the area of the film, A. Thus F = σA, where σ is the surface tension and is constant at a fixed temperature. Consequently it is possible to obtain analogue solutions to mathematical problems that require the minimization of surface area.
Consider the simple problem of finding the minimum area contained by a circular ring: it is well known that the area is a circular disc contained by the ring. An analogue solution to this problem can be obtained by dipping a ring into a bath of soap solution; when the ring is withdrawn, a soap film surface will form bounded by the ring, and when it has come to thermodynamic equilibrium it will have the shape of the disc. In practice, thermodynamic equilibrium of the surface configuration is reached as soon as the film has come to rest, in only a few seconds.
A problem that has not been solved analytically is that of linking a number of points, in a plane, by the shortest path. This problem has practical applications—for example, the linking of a number of towns by the shortest road, water pipe, or cable. The soap film provides a simple analogue mechanism for solving these minimum-path problems. They have become known as Steiner problems, after the 19th-century mathematician Jacob Steiner, who made a study of them, but it was the mathematician Richard Courant, in the 1940s, who popularized the analogue approach to the solution of these problems.
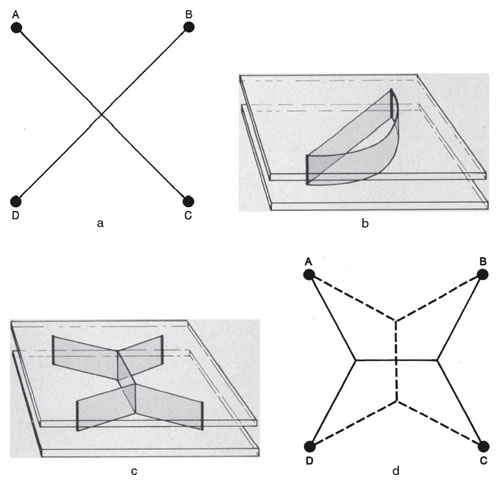
Consider the particular problem of linking four towns by the shortest road. For convenience let the towns, say A, B, C, and D, be arranged at the corners of a square. We might conjecture, on the grounds of symmetry, that the shortest roadway system joining all four towns is the X-shape given in Figure 1a, which has a length 2(2)1/2 for a square with sides of unit length. The verification or nullification of this conjecture is best examined using the analogue approach.
Before attempting to solve the four-town problem using the minimum-area property of soap films, we should first look at the one minimization problem for which the solution is well known—finding the shortest path linking two points. The solution, of course, is the straight line joining the two points. Consider two parallel Lucite plates connected by two pins perpendicular to the plates (Figure 1b).
After this system is dipped into soap solution a soap film will form between the plates, beginning on one pin and ending on the other. By symmetry it will be perpendicular to the plates and bounded, from above and below, by the plates. It will have the form of a tape of constant width, and its area will be proportional to the length of the film. When it reaches equilibrium, its area, and consequently its length, will be minimized; hence the final shape of the film joining the pins will be a straight line. Figure 1b shows a curved film while it is still not in equilibrium and the straight-line film after reaching equilibrium.
Similarly, in order to solve the problem of the four towns, we must construct two parallel Lucite plates joined by four pins perpendicular to the plates. The pins should represent, to scale, the four towns. When the plates are dipped into the soap solution, the soap film will take up the minimum area and will be in the form of a tape, of constant width, connected to all four pins (towns). The area of the film will be proportional to its length, and the minimum area will correspond to the minimum length of the film (Figure lc). At the two places where three soap films intersect, the angle between adjacent films is 120 degrees. The total length of roads is thus [1+(3)1/2 ]—shorter than the X-system length of 2(2)1/2. Because the towns are at the corners of a square, another possible solution can be obtained by rotating the first analogue solution through 90 degrees (Figure 1d).
The method of solution of the general problem of linking n towns by the shortest path is now clear. Two parallel plates are constructed and joined by pins perpendicular to the plates so that they represent, to scale, the positions of the n towns. The equilibrium soap film formed between the plates will have the minimum length, and the solution will always consist of a number (greater than or equal to zero) of intersections with three films meeting at 120 degrees, as with the four-town problem. Physically this is a consequence of three equal forces, caused by surface tension at the intersection, being in equilibrium. The soap film system will always be connected to every pin, and only one or two films can be joined to any one pin. In the latter case, the angle between the films will be greater than or equal to 120 degrees.
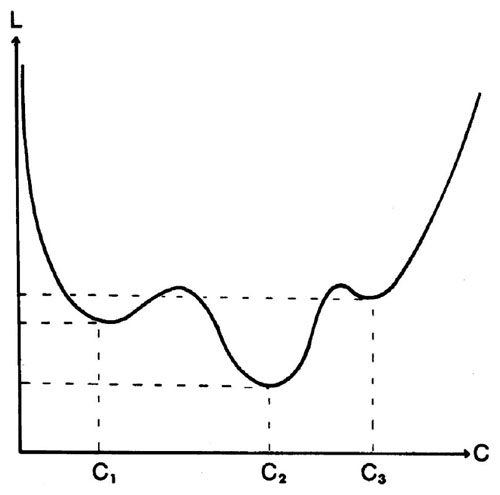
A problem may well have more than one mathematical minimum, and the soap film will always take up one of these minima. All the minimum configurations must be determined in order to pick out the shortest one. Each minimum will correspond to an equilibrium configuration of the soap film. The different configurations can be obtained by first producing one of the equilibrium configurations and then blowing onto the soap film to perturb it so that it jumps into another equilibrium configuration. In this way all the minimum configurations can be determined. Figure 2 shows the possible variation of length of the film, L, as the shape of the configuration is altered, and the length passes through all the minimum configuration as some parameter, C, is altered. It is possible to change the shape of the film by passing through nonequilibrium configurations to equilibrium configurations, which correspond to C1, C2, C3, etc.
There is no way of determining the total number of minimum configurations. By perturbing the soap film or redipping the plates in many different ways we hope to obtain all the equilibrium configurations, and during this process we often obtain the same configuration several times. The only information available for the n -town problem is the knowledge that there can be at most ( n – 2) intersections, and there may well be a smaller number.
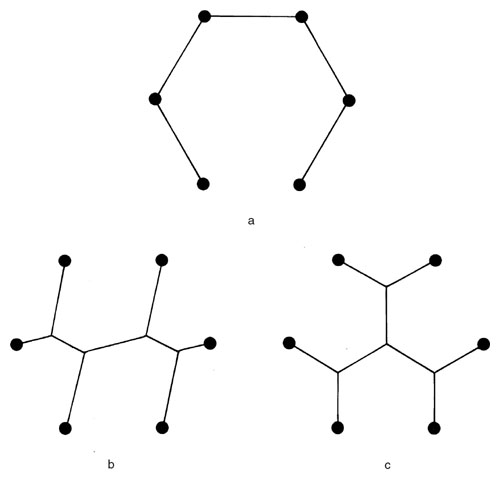
An example of a problem with several minima is the determination of the smallest minimum length of path linking six points at the corners of a regular hexagon. There are three minimum configurations (Figures 3a, b, c). Figures 3b and 3c each contain four intersections in which three films meet at angles of 120 degrees; the smallest minimum (Figure 3a) can be determined by calculation using the 120-degree property, or by measurement. Figure 3a is an example of a case in which two films meet, at a single pin, with the limiting angle of 120 degrees.
If the hexagon of pins were distorted slightly, so that it is no longer regular, maxima similar to Figures 3b and 3c would be obtained with four intersections. Figure 3a, however, would alter. Those vertices with angles that are less than 120 degrees, and previously had two films, would form intersections near the pins and have only one film joined to the pin. Figure 3a would remain the shortest minimum configuration.
We have assumed throughout that the pins have zero diameter. In practice they will have a small diameter which can produce errors: A pin of finite diameter can cause two films to meet at the pin inclined at less than 120 degrees. This is not possible for pins of zero diameter. To obtain the same configuration as in the zero-diameter case it is necessary to perturb the films by blowing onto them to make them coalesce into one film. The only difference now between the finite and zero-diameter case is the exclusion of the soap film from the region of the pin. For calculations and measurements one can extend the lengths to the center of the pins and hence obtain the same results as those for a system with pins of zero thickness.
It is possible to take the curvature of the Earth into account in solving this problem by constructing two concentric spherical shells connected by pins perpendicular to the surfaces. Again, the pins represent the towns. The lengths of the curved segments of soap film that form between the spherical shells will be proportional to the area of the soap film. The length of the film is measured along the surface of one of the spherical shells, and consequently the minimum area of the soap film will correspond to the minimum length of path connecting the “towns.”
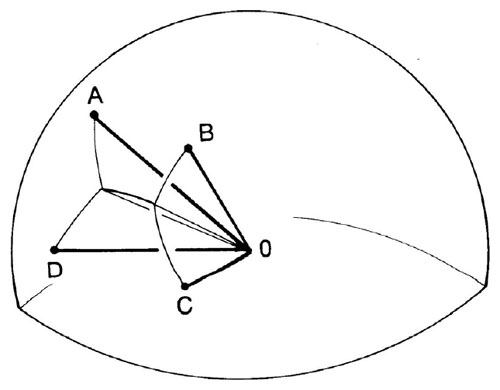
A more convenient analogue system consists of only one shell, as shown in Figure 4. From the center of the sphere, of which the shell forms part of the surface, wires radiate to points on the surface of the shell at which the “towns” are situated. The soap film that forms between the wires and the shell consists of segments whose area is proportional to their length measured along the surface of the spherical shell. Once again the minimum area will correspond to minimum length.
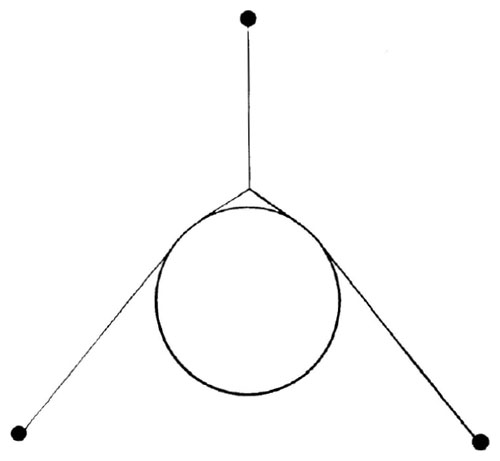
We can also take account of constraints that exclude the roadway from a particular region. For example, for a three-town problem which requires that the roadway system exclude the region of a lake at the center, a circular hole is drilled, to scale, in the Lucite plates to represent the lake, and the three pins that represent the towns are inserted. The soap film can be made to avoid the circular lake by physically breaking any soap film that forms in the hole. In the resulting shape, the roadways go around the lake and form a three-way intersection (Figure 5). An obstacle of any shape can be dealt with in this way.
The Steiner problem involves two dimensions, but it is also possible to solve three-dimensional problems using the minimum-area property of soap films. An important problem, which was solved analytically in the 18th century, is the determination of the minimum area contained by two separated coaxial rings. Each ring is arranged perpendicular to the axis. The surface is a catenary—the curve produced by a chain fixed at both ends—of revolution, providing that the distance between the rings is less than a certain value. As the distance between the rings is increased, the minimum of the catenary moves toward the axis of the rings and finally breaks up into two discs bounded by each of the rings—this is known as the Goldschmidt discontinuous solution. The catenary of revolution and the Goldschmidt solution can be demonstrated using a soap film bounded by the two rings. Mathematicians consider this problem to be one dimensional because the equation defining the surface depends on one independent variable.
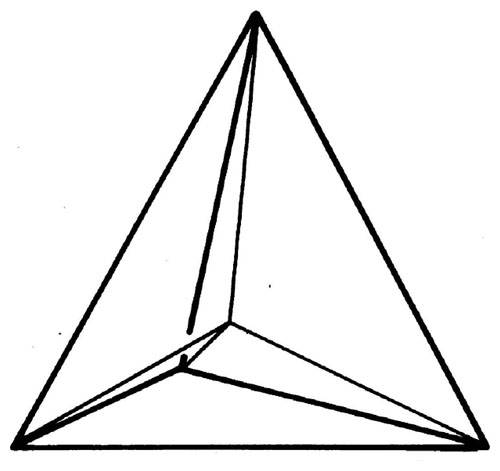
Consider the truly three-dimensional problem of determining the minimum area contained by the six edges of a tetrahedral framework. The surface is not immediately obvious; we might guess that it would consist of three triangular plane surfaces covering three faces of the tetrahedron. The analogue solution can be obtained by dipping the tetrahedral framework into soap solution, shown diagramatically in Figure 6. The soap film forms a series of plane surfaces, each of which begins at an edge of the tetrahedron and meets all the other surfaces at the center of the tetrahedron. From each vertex there is a line of soap film formed by the intersection of three planes of film. The angle between adjacent planes is 120 degrees, as was the case for the two-dimensional problem, and the four lines meet at the center of the tetrahedron and intersect each other at an angle of 109 degrees 28 minutes. Figure 7a is a photograph of this minimum surface. The horizontal interference fringes in the surface of the soap film are due to the wedge-shaped cross-section of the film which is produced by the draining away of the water in the film. The thickness of the film is comparable to the wavelength of light and hence gives rise to interference fringes.
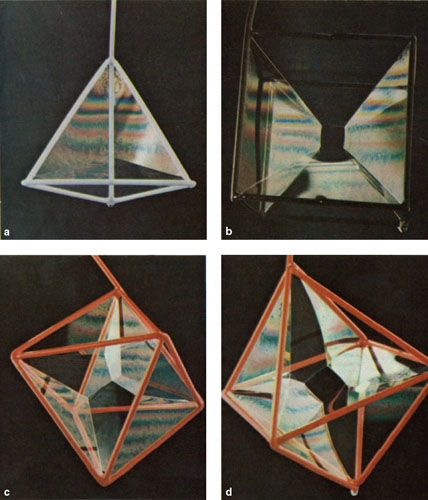
For a cubic framework we might conjecture that the minimum surface would be a set of planes, each beginning at an edge and meeting at a point at the center. However, if this did occur, the surfaces would not intersect at 120 degrees; Figure 7b shows the actual minimum surface. A “square”-shaped surface at the center of the cube ensures that the film surfaces always intersect at 120 degrees and the lines of soap film meet at 109 degrees 28 minutes. The central surface has curved edges that intersect at angles of 109 degrees 28 minutes, and the “square” can occur in a direction parallel to any of the faces of the cube. Perturbing the film, by blowing onto it, will cause the “square” to flip over to another direction. Formation of this surface at the center of the cube could have been conjectured from the two-dimensional result for the four-town problem.
The minimum surface solution bounded by the edges of an octahedron has many properties in common with the two-dimensional solution of the hexagon of points. One of several different minimum surfaces—the surface with the highest symmetry (Figure 7c) —contains four lines that meet at the center of the octahedron, as was the case for the tetrahedron. Figure 7d shows one of the other minima, which has a square in the center, similar to that in the cubic framework. (Several others are discussed in detail in Wolf 1968.)

More general mathematical problems can be investigated by including an air bubble inside the various frameworks. The free energy will contain additional terms that take account of the air in the bubble, but the minimization principle can be extended to take into account these additional terms. As the configuration of the surface is altered, the equilibrium configuration will correspond to a minimum value of the total free energy. The equilibrium configuration will, of course, depend on the quantity of air trapped in the bubble. The resulting configurations for the tetrahedron, cube and octahedron are shown in Figure 8. In each case the trapped bubble has the symmetry of the framework.
These three-dimensional solutions, with and without bubbles, were first obtained by the blind Belgian physicist Joseph Plateau (1801–1883). Many mathematicians have attempted to obtain analytic solutions to these “Plateau” problems, but no general analytic solution has been obtained, despite considerable effort. Mathematicians would like to be able to prove the existence of solutions to these three- dimensional minimization problems, in the most general case, before attempting to obtain analytic solutions. As far as the physical scientist is concerned, this is a mathematical detail— he can demonstrate the existence of a solution using soap films. An important step forward was made in the 1920s by the mathematician Jesse Douglas, for which she received the Fields medal.
More recently a number of mathematicians—Jean Taylor, Frederick J. Almgren, Jr., and Enrico Bombieri— have made important contributions to this subject; however, the end of the search for a general existence proof is not in sight. The state of mathematical knowledge of the subject has been summarized recently in the popular articles of J. C. C. Nitsche (1974) and L. A. Steen (1976). Nitsche has written that “mathematical existence proofs for surface systems” such as those depicted in Figure 7 “as well as the investigations of the nature and the regularity of the branch lines are still in their infancy.”
Editors’ Note: These letters appeared in the January–February 1977 issue of American Scientist. in response to the 1976 feature "The Soap Film: An Analogue Computer." The feature has been reprinted (above) as part of American Scientist's centennial celebrations.
To the Editors:
Certain impressions left in the article “The Soap Film: An Analogue Computer,” by Cyril Isenberg (Am. Sci. 64:514-18, Sept. 1976), should be corrected. First, Dr. Isenberg’s comments on the Steiner problem (linking a number of points in a plane by the shortest path) are misleading. He says that the “problem has not been solved analytically, and that “there is no way of determining the total number of [locally] minimum configurations.” But in fact there is an explicitly computed maximum for the number of such solutions (Gilbert and Pollack, SIAM J. Ap. Math. 16:11,1968), they can all be listed, and the major computing problem is simply to find out which of these possible local minima is the smallest (this, however, is an NP complete problem, and thus the difficulty of doing this comparison may rise exponentially with the number of points). A good reference is L. Steen, Science News 109:298-301,1976. More important, Dr. Isenberg’s comments on the mathematical aspects of the general soap film problem are several years out of date. In fact, the existence of solutions to the general soap film and bubble problem was proved by F. J. Almgren, Jr., and the nature and smoothness of their branch lines was proved by me; these results were published in mathematical journals early this year and were discussed in the July 1976 issue of Scientific American; they have been known informally by the mathematical community since about the time of publication of Nitsche’s article quoted by Dr. Isenberg (1974) and were outlined in the article by Steen cited by Dr. Isenberg (the correct reference is Science News 108:186-7, 1975). Also, note that the bubble in Figure 8c does not have the symmetry of its boundary.
Jean E. Taylor
Department of Mathematics
Rutgers University
New Brunswick, NJ
Dr. Isenberg replies:
In my article I implied that the general case of the Steiner problem, with n points, could not be written down explicitly with pen and paper. I was comparing the analogue method based on soap film with results that can be obtained with pen and ink. I excluded the use of high-speed digital computers. At the time I visited the United States in January of last year Dr. Taylor’s publication was not known to me, and I could not have been aware of her results prior to publication. I will look forward to seeing the explicit solutions to the Plateau surfaces and bubbles contained by frameworks.
Click "American Scientist" to access home page
American Scientist Comments and Discussion
To discuss our articles or comment on them, please share them and tag American Scientist on social media platforms. Here are links to our profiles on Twitter, Facebook, and LinkedIn.
If we re-share your post, we will moderate comments/discussion following our comments policy.