
This Article From Issue
July-August 2009
Volume 97, Number 4
Page 334
DOI: 10.1511/2009.79.334
THE GREAT EQUATIONS: Breakthroughs in Science from Pythagoras to Heisenberg. Robert P. Crease. 315 pp. W. W. Norton and Company, 2008. $25.95.
The growing stream of popular books on science is fundamentally an encouraging development: More and more people want to learn about science. As these books proliferate, they also raise interesting questions about how best to address and meet this large audience. We now have several books that enumerate and describe the greatest experiments or equations, so new books in this field rightly need to add something, not repeat the same stories ad infinitum. Robert P. Crease, who is chair of the Philosophy Department at Stony Brook University as well as a historian at the Brookhaven National Laboratory and who writes a regular column for Physics World, has already written five well-regarded books about physics and physicists. In his new book, The Great Equations, Crease makes excellent use of his philosophical background and his long-standing interest in the history of science.
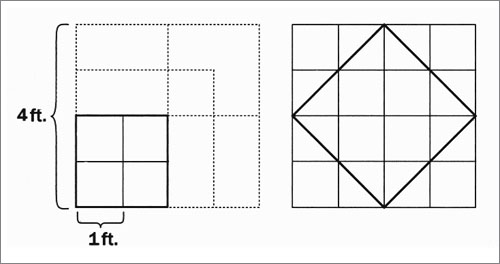
From The Great Equations.
Not surprisingly, each of the equations he discusses is a deservedly famous and long-established classic: the Pythagorean theorem; Isaac Newton’s second law of motion and his equation of universal gravitation; Leonhard Euler’s famous equation relating e, i, π, 1, and 0; the second law of thermodynamics; James Clerk Maxwell’s equations; Albert Einstein’s E=mc2 and his field equations for general relativity; Erwin Schrödinger’s wave equation; and Werner Heisenberg’s uncertainty relation (which is an inequality, Crease notes, not strictly an equation). Crease provides a succinct and well-crafted account of the history of each of these celebrated equations. He often draws attention to their collective authorship rather than treating them as the sudden or purely idiosyncratic inspiration of a single individual, as admiring myths would have it. Crease emphasizes the slow development of these ideas and the historic matrix within which the equations emerged, often through paradox and perplexity. Thus, in his treatment, the seminal equations are not mysterious hieroglyphs but summations and distillations of whole trains of thought that the reader is invited to follow and understand.
Crease’s writing is intelligent and engaging throughout; although he presents the arguments in a nontechnical way, he does not dumb things down. His explanations have great conceptual clarity and are highly accurate. Only a few passages are problematic.
In the chapter on E=mc2, readers might be misled by this statement: “Light acts more like sound. Sound always travels at the same speed (about 1,100 feet a second in air), regardless of how fast its source travels.” Although the speed of sound depends only on properties of the medium, in important ways light does not act like sound. The passage of sound does depend on the velocity of the source with respect to the medium, but the passage of light depends only on the relative speed of observer and source: Unlike sound, light can travel in a vacuum without a medium. Thus, the Doppler shift observed using light differs from the Doppler shift of sound.
At the beginning of his 1905 relativity paper, Einstein mentions “asymmetries” that seem to emerge from Maxwell’s electrodynamics “as usually understood at present.” Crease interprets “asymmetries” as “eccentric results.” A reader might well be puzzled by what was “eccentric” about them or why (as Crease puts it) they “seem to be an artifact of our theories rather than a part of the world.” It would have helped to explain (if only in the notes) that Einstein was using the term asymmetries to refer to the results of simple thought experiments suggested by Maxwell’s equations—experiments in which, for example, a magnet in motion near a stationary coil of wire seems to produce different results than does a coil in motion near a stationary magnet. According to the principle of relativity, both these cases should produce the same observable result; thus such a disagreement would signal an “asymmetry.”
For the benefit of readers uneasy with equations, the book could have addressed further the historical emergence of equations as conceptual tools. Crease is right to emphasize the wonder implicit in “1+1=2.” As Socrates long ago asked, why exactly is this true? How does two ever come from one? Algebraic equations, with all their mysterious symbols, are even more perplexing. Crease touches on the role of René Descartes in the story of equations, but I wish he had also included the 16th-century French attorney, code breaker and mathematician François Viète, who introduced alphabetic symbols for the unknowns and coefficients of equations. Knowing something more of this history, readers might be reassured to realize that any perplexities they have reflect the inherent difficulty of the whole concept of equations, which required radical conceptual innovations that still challenge the mind.
These cavils notwithstanding, Crease has made sound choices among a rich array of possibilities; not everything can be covered in a short book. And his exposition has many really felicitous passages. For instance, he emphasizes the related concepts of invariance and covariance. Something can “look different from different perspectives,” he notes. “Yet all the time I am seeing the same object. Invariance therefore involves understanding unity as it shows itself in changing appearances.” As Crease explains it, covariance is “simply part of what we mean by objectivity.” Here Crease’s philosophic awareness helps the reader overcome the common misapprehension that relativity theory means that “everything is relative.”
Crease’s historical understanding is no less sensitive and well-informed. He rightly presents the origins of quantum theory in the context of thermodynamics and statistical physics, avoiding the widely repeated (but historically unfounded) claim that the introduction of the quantum came in direct response to a hypothetical “ultraviolet catastrophe,” a contradiction between classical mechanics and electrodynamics presumed to have been known to Max Planck and Einstein during the period from 1900 to 1905. As Martin Klein pointed out, this “catastrophe” was only mentioned in the literature after 1911. Crease is also careful to avoid overstating the importance of the Michelson-Morley experiment for Einstein. As Gerald Holton noted, Einstein always denied that he had relied on that experiment as he formulated special relativity theory; rather, it was the conceptual “asymmetries” mentioned earlier that really set him thinking.
Too many writers of popular books on science succumb to the temptation to resort to startling or outré analogies, such as the wild mystical comparisons that mar some accounts of quantum theory. I admire Crease’s deft and ingenious comparisons, which are fresh and striking, not forced or bizarre. Thus, he compares “the ‘intermediate kind of reality’ that Heisenberg said was the price one had to pay to have quantum phenomena” with
the funny kind of incomplete, semiabstract reality that scripts or scores have—they are programs, as it were, for real things in the world (the produced play, the performed music) that require adding a context, and decisions about that context affect the whole of the abstract object. It brings back the role of human purposes and decisions that Newton left out.
Or again, describing the difference between crank theories and the (sometimes even more conceptually bizarre) genuine article, Crease draws attention to the way cranks tend to use “either no equations or a small number treated like fetish objects.” Also, the equations appear unaccompanied, he says, rather than being embedded in extensive arguments. In crank outpourings, “the role of the equation in the paper is like that of a musical instrument that someone carries around without ever playing.”
These examples give something of the flavor of this admirable book. Crease’s insightful and clear exposition, memorable comparisons and fine writing, and above all his philosophic thoughtfulness, make The Great Equations both richly instructive and delightful.
Peter Pesic teaches at St. John’s College, Santa Fe. He is the author of Labyrinth: A Search for the Hidden Meaning of Science (2000), Seeing Double: Shared Identities in Physics, Philosophy, and Literature (2002), Abel’s Proof: An Essay on the Sources and Meaning of Mathematical Unsolvability (2003), and Sky in a Bottle (2005), all published by The MIT Press. His new edition of Hermann Weyl, Mind and Nature: Selected Writings on Philosophy, Mathematics, and Physics, was published by Princeton University Press in April 2009.

American Scientist Comments and Discussion
To discuss our articles or comment on them, please share them and tag American Scientist on social media platforms. Here are links to our profiles on Twitter, Facebook, and LinkedIn.
If we re-share your post, we will moderate comments/discussion following our comments policy.