American Scientist

Avogadro's number, N_A, is the fundamental physical constant that links the macroscopic physical world of objects that we can see and feel with the submicroscopic, invisible world of atoms. In theory, N_A specifies the exact number of atoms in a palm-sized specimen of a physical element such as carbon or silicon.

The name honors the Italian mathematical physicist Amedeo Avogadro (1776-1856), who proposed that equal volumes of all gases at the same temperature and pressure contain the same number of molecules. Long after Avogadro's death, the concept of the mole was introduced, and it was experimentally observed that one mole (the molecular weight in grams) of any substance contains the same number of molecules. This number is Avogadro's number, although he knew nothing of moles or the eponymous number itself.

Today, Avogadro's number is formally defined to be the number of carbon-12 atoms in 12 grams of unbound carbon-12 in its rest-energy electronic state. The current state of the art estimates the value of N_A , not based on experiments using carbon-12, but by using x-ray diffraction in crystal silicon lattices in the shape of a sphere or by a watt-balance method. According to the National Institute of Standards and Technology (NIST), the current accepted value for N_A is:

N_A = (6.0221415 ± 0.0000010) × 10²³

This definition of N_A and the current experiments to estimate it, however, both rely on the precise definition of a gram. Originally the mass of one cubic centimeter of water at exactly 3.98 degrees Celsius and atmospheric pressure, for the past 117 years the definition of one gram has been one-thousandth of the mass of "Le Gran K," a single precious platinum-iridium cylinder stored in a vault in Sèvres, France. The problem is that the mass of Le Gran K is known to be unstable in time. Periodic cleanings and calibration measurements result in abrasion of platinum-iridium and accretion of cleaning chemicals.

These changes cannot be measured exactly, simply because there is no "perfect" reference against which to measure them—Le Gran K is always exactly one kilogram, by definition. It is estimated that Le Gran K may have changed about 50 micrograms—that is, roughly by about 150 quadrillion (1.5 × 10¹⁷) atoms—since it was constructed. This implies that by current measurement conventions, the mass of a single atom of carbon-12 is changing in time, whereas modern theory postulates that it remain constant.

Illuminating c

A similar predicament concerning the speed of light existed until two decades ago. Although a basic premise of modern physics is that the speed of light is constant, from the early 1600s until the latter part of the 20th century, the official definition of the speed of light also varied with time.

The empirical estimates for the speed of light relied on the definition of a second at the time of the experiment (for example, in recent times, via the resonant frequency for the hyperfine transition in cesium-133, where the 10-digit integer 9,192,631,770 hertz defines one second), and they relied on the definition of a meter, which had evolved from being one ten-millionth of the distance from the Equator to the North Pole on the meridian that passes through Paris, to being the exact length of another single platinum-iridium artifact, a unique official meter stick. But as with Le Gran K, the length of the official meter-stick artifact was also changing with time, implying that the official value for the speed of light was changing with time.

On October 21, 1983, the roles of the constants expressing the speed of light and the length of one meter were reversed when the Seventeenth International Conference on Weights and Measures defined the meter to be the distance traveled by light in a vacuum in exactly 1/299,792,458 of a second. That eliminated the continuously changing official value for the speed of light, and since 1983 the distance one meter has been approximated experimentally using these fixed values for the speed of light and the second. The new numerical value chosen for c was the closest integer to the experimentally observed value, and since it was accurate to nine digits, was well within the range of experimental errors of laboratory equipment. More important theoretically, the new fixed definition of c eliminated the necessity of the artifact meter stick forever.

Farewell to Le Gran K

A similar solution can solve the dilemma of the current time-dependent definition of Avogadro's number. The idea is simply to define N_A , once and for all, as was done for the speed of light. Unlike that case, however, the range of known possible values for N_A is astronomical. Three desirable basic properties for a reasonable value for N_A help narrow the search.

First, since Avogadro's number purports to count the number of atoms in some theoretical specimen, its value should be an integer, as any schoolchild would expect. This would avoid having to interpret one-third of an atom, or worse yet, 1/p of an atom.

Second, the value chosen should be within the currently accepted range, (6.0221415 ± 0.0000010) × 10²³.

Third, the value chosen for Avogadro's number should ideally have some inherent physical significance. Since volumes of objects are measured cubically, as in cubic centimeters and cubic yards, and not spherically (for example, via volumes of spheres with unit radii or diameters), and since the current definition of Avogadro's number counts the number of atoms in a solid specimen,it is reasonable to imagine the object as being a perfect geometrical cube. That implies that the value chosen should be a perfect numerical cube.

The range of acceptable integers in the current estimate of N_A is two hundred quadrillion (2 × 10¹⁷), but within that huge range of values there are only 10 perfect cubes—from 84,446,884³ to 84,446,893³. For our purposes, any one of those 10 may be used, but the one closest to the best current estimate of Avogadro's number, and the only one accurate to within one unit in the eighth significant digit of the current best estimate, is

N_A* = 602,214,141,070,409,084,099,072 = 84,446,888³.

Our proposal is simply to define Avogadro's number, permanently, as was done with the speed of light and with the second, and to set it equal to this specific integer. If the sides of the cube of atoms were only six atoms shorter or longer, the number of atoms it contains would no longer be within the currently accepted range for Avogadro's number, since 84,446,883³ = (6.02214034+) × 10²³ and 84,446,894³ = (6.02214269+) × 10²³.

Since the shape of a volume certainly affects the numbers of molecules it can contain—extremely long, thin cylinders can contain none—it seems natural to ask that the shape of the defining volume be a cube. Of course any other solid shape could also suffice as the defining object, but using a rectangular solid or parallelpiped would require specification of three numbers: the length, width and height. Using a sphere precludes choosing an integer at all, because of the irrationality of p.

At first glance, another possible candidate for the exact value of Avogadro's number might be 602,214,150, 000,000,000,000,000, which is dead center in the current range of values. This value, however, has little physical significance. It is neither a perfect cube nor a perfect square, so no perfect geometrical cube or square of atoms could be constructed which has that exact volume or area.

Moreover, the method of simply using the most recent best estimate of N _A is not robust, unlike the methods that were used for defining fixed values for the speed of light and the second. If current experimental estimates of Avogadro's number increase the known number of significant digits by four or five places, for example, the "current best estimate" method of fixing the value for Avogadro's number would presumably also change by those same four or five digits.

The fixed values for the meter in terms of the speed of light and for the second in terms of vibrations of a cesium atom, however, were nearest-integer solutions, insensitive to further fractional refinements of the exact measurements. In exactly that same spirit, the definition of N_A* above is also a nearest-integer solution—the nearest integral side length of a cube containing Avogadro's number of atoms. As such, the value chosen is also insensitive, within one atom either way, to improved experimental estimates of N_A. The choice of an integer value for N_A* seems essential, whereas the requirement that it be a perfect cube is largely esthetic, but with practical and intuitive physical significance as well.

Squaring N_A with N_A*

Adoption of N_A* as the value for Avogadro's number would offer several advantages. With today's definition of Avogadro's number being the number of atoms in one mole of a particular element, this new fixed value for it would simply mean that the mass of a simple cube of carbon-12 atoms, exactly 84,446,888 atoms on a side, is exactly 12 grams by definition.

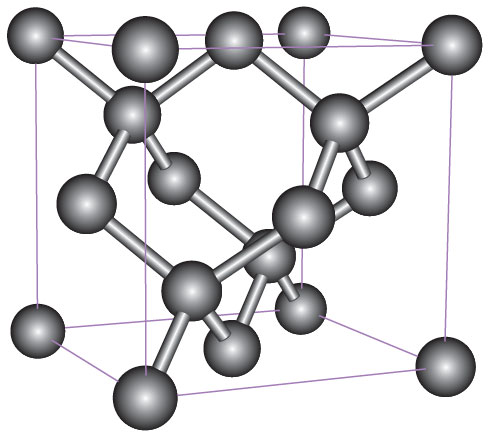
Practically speaking, however, carbon does not admit an extended simple cubic structure but does have a face-centered cubic (FCC) crystal structure in three dimensions, the same as diamond and silicon. This means that in a real physical cubic array of carbon atoms, the atoms are located not only in a simple cubic array but also at the centers of faces made by a square of four adjacent planar atoms and at certain interior tetrahedral centers of cubes made of eight adjacent corner atoms. The number of atoms in such an actual FCC array with k atoms on each edge can easily be calculated to be 8k ³-18k ²+15k-4.

Carbon-12 is special in the context of fundamental constants since, by convention, NIST uses carbon-12 to define both Avogadro's number and the basic atomic mass unit, amu. Thus, if one wanted a definition of Avogadro's number specifically tied to the actual physical FCC lattice structure of carbon-12, one could replace the earlier formula n³ = N _A by 8k ³-18k ²+15k-4 = N_A. This means that a physical FCC lattice of carbon-12 containing 42,223,444 atoms on each edge, exactly half the number of atoms on the edge of the hypothetical cube defining N_A* above, would contain exactly 602,214,108,979,663,699,470,280 atoms (8k³-18k ²+15k-4 with k = 42,223,444), which is also within the currently accepted range of values for Avogadro's number but not as close to the best estimate as is N_A*.

Of course, the instant a fixed value for Avogadro's number is chosen, there no longer would be scientific interest in constructing an exact such cube anyway, just as there has been no scientific quest to construct the perfect meter stick since 1983. Building meter-stick and gram prototypes would be left to manufacturers of precision surveying and scales equipment.

Numerically, N_A* is describable in nine digits (eight digits plus the exponent), and in that sense contains roughly the same order of magnitude of information as the fixed integers that define the speed of light and the second. Moreover, 84,446,888 (or 42,223,444) is easy to remember. Since N_A* is almost dead center within the current known range of values for N_A, it is consistent with current experimentally obtained results.

Replacing the Kilogram Standard

Le Système International d'Unites (SI), the organization that oversees measurements and standards that have been officially recognized and adopted by nearly all countries, identifies exactly seven basic units. These official units and their standards of measurement are length (meter), mass (kilogram), time (second), electrical current (ampere), thermodynamic temperature (kelvin), amount of substance (mole) and luminous intensity (candela).

Of these basic seven, which are assumed to be mutually independent, the kilogram is the only unit that is still defined in terms of a physical artifact. Not only is this definition inelegant, but it is also labor-intensive compared with the fundamental and universal definitions of the other units. Maintaining and preserving Le Gran K—cleaning and calibrating and compensating for lost platinum-iridium atoms and adsorbed contaminants—requires intensive labor and expense. This labor is duplicated scores of times around the world, where many national bureaus of standards maintain their own replicas of Le Gran K. These subordinate lesser Ks also require periodic re-calibration with the French K and with their own subordinate scale users. For these reasons, there has been considerable effort to design a method that will eliminate the need for this final SI artifact.

Using N_A* carbon-12 atoms to define 12 grams is one such solution. The two main candidates for an alternative definition of the kilogram, the silicon-lattice method and the watt-balance method, are both experimental in nature, and thus, as with the Le Gran K definition, change in time depending on the state of the art of the laboratory equipment used in the experiments.

The proposal to use N_A* also offers a distinct advantage in reducing experimental errors. Using today's methods for determining Avogadro's number requires two experiments, usually far apart in time and space: first, calibrating the scales (at the laboratory, often not in France) with Le Gran K in France; and second, running the N_A experiment. The resulting best current approximation to N_A thus compounds the errors from both experiments. Precisely the same experiments that are used to determine N_A, when viewed from the perspective of a known fixed value (say N_A*) for Avogadro's number, would now simply measure 1 gram.

For example, running the experiment with the crystal silicon sphere mentioned above would proceed in exactly the same manner as before. The total number of atoms in the sphere would be estimated the same way, but now N_A* would determine the mass of the sphere, and weighing the sphere with scales would now be calibrating the scale, not vice versa.

In conclusion, adopting this natural value N_A* for Avogadro's number would be elegant and easy and would have far-reaching and important consequences experimentally, theoretically and economically. Above all, it would eliminate forever the dependence of SI physical units on manmade objects.