A Helix with a Handle
By Fenella Saunders
Mathematicians prove the existence of a new class of minimal surfaces
Mathematicians prove the existence of a new class of minimal surfaces

DOI: 10.1511/2006.59.216
Dip a loop of wire into a soapy solution, and the film that covers the loop will be what mathematicians call a minimal surface. The soap forms such a shape because it minimizes surface tension. At any point, a minimal surface is maximally curved in one direction and minimally curved in the opposite direction, but the amount of curvature in each direction is exactly the same. As a result, each point on the surface is either a flat plane or a saddle shape, never a sharp peak or valley. But a minimal surface doesn't have to be flat or simple overall: A plane can be twisted into a parking-ramp shape called a helicoid, which mathematicians proved over two centuries ago is also a minimal surface.
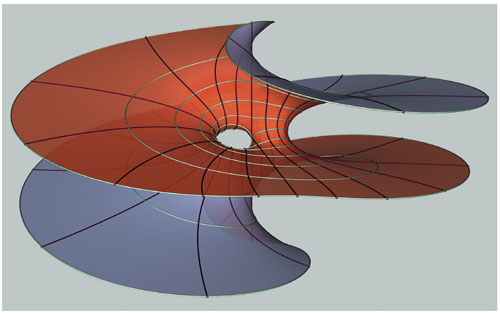
Matthias Weber
Mathematicians have proved the existence of a class of minimal surfaces that cannot be embodied by soap bubbles but can be visualized by computer simulation. This surface, called a genus-one helicoid, is a variation on a standard helicoid, but there is a tunnel through the deck of the parking-ramp spiral. When untwisted, this surface looks like a flat sheet with a coffee-mug-handle shape grafted onto it. "Think of a torus, like an inner tube," says Matthias Weber of Indiana University. "Now imagine that you puncture the torus. This results in a surface that can be stretched and deformed into the genus-one helicoid. I think that's a real mind bender."
As they reported in the November 15, 2005, issue of the Proceedings of the National Academy of Sciences, Weber, David Hoffman of Stanford University and Michael Wolf of Rice University have proven that such shapes, whether they have one or an infinite number of handles, are indeed minimal surfaces that can go on forever in all directions and never fold back to intersect themselves.
Over a decade ago, Hoffman, with Fusheng Wei, then of the University of Massachusetts at Amherst, and Hermann Karcher of the University of Bonn in Germany, had created computer simulations of such handled helicoids, but an airtight demonstration of minimal surfacehood eluded them. "Computer graphics programs enabled us to visualize these surfaces, but we couldn't bring them back into the mathematical fold," says Hoffman. "I think the information about how to solve this problem was lurking in the pictures all the time, but we just had to think about it for a long time and have the theory catch up with the evidence we had." Catching up can be hard to do: The mathematical proof takes up more than 100 pages.
An advanced understanding of minimal surfaces could be relevant to materials science; for instance, some compound polymers, such as Kevlar, have interfaces between molecules that are approximately minimal surfaces, the shape of which can influence the chemical properties of the material.
As mathematicians, Weber and his colleagues are most excited about a potentially large, new class of minimal surfaces that have not been found in nature and which no investigators had imagined could exist until recently. "It's easy to come up with one new example of a minimal surface, but this one is of a very different nature than others that have been found before," Weber said. "So it's opened a new field within the theory of minimal surfaces."
Click "American Scientist" to access home page
American Scientist Comments and Discussion
To discuss our articles or comment on them, please share them and tag American Scientist on social media platforms. Here are links to our profiles on Twitter, Facebook, and LinkedIn.
If we re-share your post, we will moderate comments/discussion following our comments policy.